Question

I need to evaluate the derivative of the function and find the answer

Answer 1

C.

General Formulas and Concepts:

Calculus

Differentiation

• Derivatives
• Derivative Notation

Derivative Property [Multiplied Constant]:
`\displaystyle (d)/(dx) [cf(x)] = c \cdot f'(x)`

Derivative Property [Addition/Subtraction]:
`\displaystyle (d)/(dx)[f(x) + g(x)] = (d)/(dx)[f(x)] + (d)/(dx)[g(x)]`

Basic Power Rule:

1. f(x) = cxⁿ
2. f’(x) = c·nxⁿ⁻¹

Derivative Rule [Quotient Rule]:
`\displaystyle (d)/(dx) [(f(x))/(g(x)) ]=(g(x)f'(x)-g'(x)f(x))/(g^2(x))`

Explanation:

Step 1: Define

Identify

`\displaystyle f(t) = (8t + 2)/(4t - 1)`

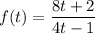
Step 2: Differentiate

1. Derivative Rule [Quotient Rule]:
   `\displaystyle f'(t) = ((8t + 2)'(4t - 1) - (8t + 2)(4t - 1)')/((4t - 1)^2)`
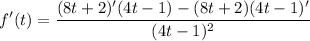
2. Basic Power Rule [Derivative Properties]:
   `\displaystyle f'(t) = (8(4t - 1) - 4(8t + 2))/((4t - 1)^2)`
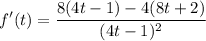
3. Simplify:
   `\displaystyle f'(t) = (-16)/((4t - 1)^2)`

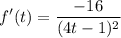
Step 3: Evaluate

1. Substitute in point [Derivative]:
   `\displaystyle f'(3) = (-16)/([4(3) - 1]^2)`
2. Simplify:
   `\displaystyle f'(3) = (-16)/(121)`

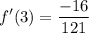
Topic: AP Calculus AB/BC (Calculus I/I + II)

Unit: Differentiation