Question

Please help me . sooooooooooon

Answer 1

[I didn't finish this and I have to go, so I'll post what I have so far and come back in a six or so hours.

Let's understand these equations a bit before we start.

The first is almost the the Law of Cosines with triangle with sides
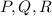
`P,Q,R` with the supplement of
`a` opposite R. The plus sign means have use the supplement.

The second is the near Law of Cosines with sides
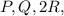
`P,Q,2R,` with the supplement
`b` opposite
`2R`.

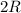
So this says given two triangles with the two pairs of sides congruent, and the other side twice as big in the second, show the supplements of the angles opposite the different sides satisfy


`2 \cos ( \frac a 2 ) = \cos ( \frac b 2 )`

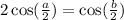
It doesn't really matter if we understand the geometry; it's an algebra quetsion really. Yes I'm using a and b not alpha and beta, easier to type.


`R^2 = P^2 + Q^2 + 2 PQ \cos a`


`\cos a = (R^2 - P^2 - Q^2)/( 2 PQ )`

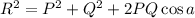
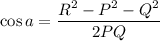
We need the half angle; let's put it in using the cosine double angle formula.


`\cos a = 2 \cos ^2 \frac a 2 - 1`


`2 \cos ^2 \frac a 2 = 1 + \cos a`


`2 \cos ^2 \frac a 2 =(R^2 - P^2 - Q^2 + 2PQ)/( 2 PQ)`


`2 \cos ^2 \frac a 2 =(R^2 - (P- Q)^2)/( 2 PQ )`

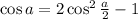
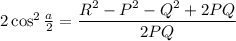
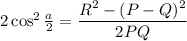
Analogously,


`2 \cos ^2 \frac b 2 =(4R^2 - (P- Q)^2)/( 2 PQ )`

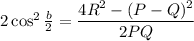
Dividing,


`( 2 \cos ^2 \frac b 2)/(2 \cos ^2 \frac a 2) =(4R^2 - (P- Q)^2)/(R^2 - (P- Q)^2)`


`{\cos ^2 \frac b 2} = (\cos ^2 \frac a 2 ) (4R^2 - (P- Q)^2)/(R^2 - (P- Q)^2)`

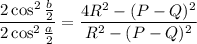
We'd be home if that pesky difference term (P-Q)^2 wasn't there, but it is. Gotta go.