Question

A data set includes 110110 body temperatures of healthy adult humans having a mean of 98.098.0degrees°f and a standard deviation of 0.740.74degrees°f. construct a 9999​% confidence interval estimate of the mean body temperature of all healthy humans. what does the sample suggest about the use of 98.6degrees°f as the mean body​ temperature?

Answer 1

I assume that says
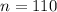
`n=110` and
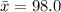
`\bar x = 98.0` and
`\sigma = 0.74`.

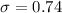
It doesn't really tell us if this is the standard deviation of the individual samples or of the sample average. Since they're related by a factor of
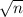
`√(n)`, around ten, some common sense tells us this is the standard deviation of the individual samples.

So we can conclude our sample mean has a standard deviation


`s = ( \sigma )/( \sqrt n ) = (.74)/( √(110)) = 0.0705562`

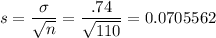
and that
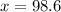
`x=98.6` is


`z = ( x- \bar x)/(s) = (98.6 - 98.0)/(0.0705562 ) = 8.5`

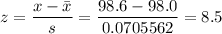
That's a huge z score, 8.5 standard deviations away. 110 is big enough we can just use the normal distribution and not worry about t distributions.

We can conclude the 98.6 is very unlikely to be the true mean or close to the true mean of human temperatures.