Question

A ball is propelled upwards from ground level. After t seconds it's height in feet is a function defined by f(t)=-16t sq +96t.

After how many seconds will it reach a height of 128ft.

Answer 1

well, we know the height is f(t), and we know that f(t) = -16t² + 96t, with "t" being the seconds.

so, when will it reach 128ft? namely, what is "t" when f(t) = 128?


`\bf \stackrel{f(t)}{128}=-16t^2+96t\implies 16t^2-96t+128=0\\\\\\ 16(t^2-6t+8)=0 \\\\\\ t^2-6t+8=0\implies (t-2)(t-4)=0\implies t= \begin{cases} 2\\ 4 \end{cases}`

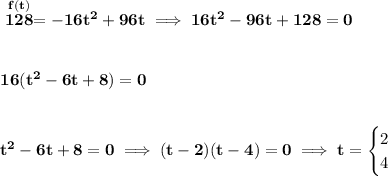
well, so it reaches it twice, check the picture below.

at 2 seconds on its way up, and at 4 seconds on its way down.