Question

What is the slope of a line that is parallel to the line y = -1/5?

Answer 1

Parallel lines have, by definition, the same slope:


`L_1 \parallel L_2 \implies m_1=m_2`

Therefore:

`m_2=m_1=-(1)/(5)`

Answer 2

Slope of line parallel to given line is 0.

Explanation:

Given Equation of the line ,

`y=(-1)/(5)`

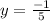
We need to find slope of line which is parallel to given line.

We know that When line are parallel then their slope are equal.

So, Slope of line which is parallel to given line is equal to the slope of given line.

We find slope of given line by comparing it with slope intercept form of the line.

Slope-intercept for, y = mx + c where m is slope of the line.

Given line ,
`y=(-1)/(5)`

`y=0x+(-1)/(5)`

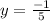
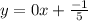
On comparing, we get slope of given line is 0

Therefore, Slope of line parallel to given line is 0.