Question

A triangle drawn on a map has sides that measure 15 cm, 8 cm, and 10 cm. The shortest of the corresponding real-life distances is 99 km. Find the longest of the real-life distances.

Answer 1

The shortest side on the map is 8 cm. This IN real-life distances is 99 km. To find the ratio, we need to change 99 km to cm.

`99 \: km * (1000 \: m)/(1 \: km) * (100 \: cm)/(1m) \\ 99 \: km = 9900000 \: cm`
This is a ratio of

`8 * r= 9900000 \\ r = (9900000)/(8) \\ r = 1237500`
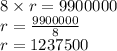
Now that we have the ratio, we can find the longest side of the triangle.

The longest side of the triangle is 15 cm.

`15 * 1237500 = 18562500 \: cm`
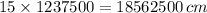
In km, this is

`18562500 \: cm * (1m)/(100cm) * (1km)/(1000m) \\ 18562500 \: cm = 185.625 \: km`
The longest side of the real-life triangle is 185.625 km, or 185 5/8 km