Given equation ---›
2x + 7y = 4 (1st equation)
-4x - 3y = 14 (2nd equation)
Multiply 1st equation by 2 and then add it by second equation

So,
We get the value of y = 2
Now,,
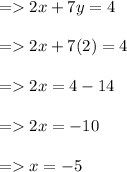
Therefore value of x = -5.
Expressing the answer as an ordered pair in the format (a,b) => (-5, 2)