Question

The time t required to drive a centain distance varies inversely with the speed r. If it takes 2 hours to drive the distance at 30 mines per hour how long will it take to drive the same distance at 50 miles per hour

Answer 1

An inversely proportional equation for our set of circumstances looks like this:
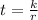
`t= (k)/(r)`. We have a t value of 2 and an r value of 30, so we will sub those values in and solve for k, the constant of variation.
`2= (k)/(30)`. Multiply both sides by 30 to get that k = 60. Now we need to find t when r = 50. We can do this now because we have a value for k.
`t= (60)/(50)` and t = 1.2 hours.