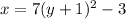I'm assuming by the way those points lie in the coordinate plane that this is a parabola that opens to the right, a y^2 parabola. Our x and y coordinates are (4,0), and the h and k of the vertex are (-3, -1). The standard form for this parabola is
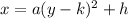
. We need to find a. Filling in accordingly,
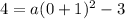
. Simplifying a bit we get 4 = a - 3. a = 7. The parabola, then, has the equation