Question

What is the length of EF in the right triangle below?

Answer 1

(B)
`EF=√(120)`

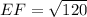
Explanation:

Given: From the figure, it is given that in triangle DEF, DE=13 and DF=7

To find: The value of EF.

Solution: It is given that From the figure, it is given that in triangle DEF, DE=13 and DF=7, therefore using Pythagoras theorem, we have

`(DE)^2=(DF)^2+(EF)^2`

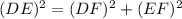
Substituting the given values, we get

`(13)^2=(7)^2+(EF)^2`

`169=49+(EF)^2`

`169-49=(EF)^2`

`120=(EF)^2`

`EF=√(120)`

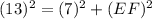
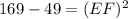
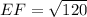
Thus, the value of EF is
`=√(120)`.

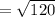Hence, option B is correct.

Answer 2

Length of E F

a² + b² = c²
a² + 7² = 13²
a² + 49 = 169
a² = 169 - 49
a² = 120
a = √120

Answer = √120