Question

What is the length of the hypotenuse of the triangle below?

Answer 1

C=-18 that should be the answer if im right i hope that helped

Answer 2

Answer: The correct option is (A) 18 units.

Step-by-step explanation: We are given to find the length of the hypotenuse of the triangle in the figure.

Given in the figure that,

lengths of the two legs of the right-angled triangle are each 9√2 units,

So,

`\textup{perpendicular, }p=9\sqrt2~\textup{units},\\\\\textup{base, }b=9\sqrt2~\textup{units}.`

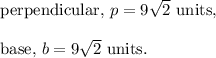
Length of the hypotenuse, h = ?

Using Pythagoras theorem, we have from the given right-angled triangle,

`\textup{hypotenuse}^2=\textup{perpendicualr}^2+\textup{base}^2\\\\\Rightarrow h^2=p^2+b^2\\\\\Rightarrow h^2=(9\sqrt2)^2+(9\sqrt2)^2\\\\\Rightarrow h^2=162+162\\\\\Rightarrow h^2=324\\\\\Rightarrow h=18~\textup{units}.`

Thus, the length of the hypotenuse is 18 units.

Option (A) is correct.