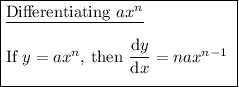Question

The graph of the function y = x^3 + ax^2 + b has a local minimum point at (4, -11). Find the coordinates of the local maximum. Answer: (0, 21)

Answer 1

(0, 21)

Explanation:

Stationary points occur when the gradient of a graph is zero.

Therefore, to find the x-coordinate(s) of the stationary points of a function, differentiate the function, set it to zero and solve for x.

Differentiate the given function:

`\begin{aligned}y & = x^3+ax^2+b\\\implies \frac{\text{d}y}{\text{d}x}&=3 \cdot x^(3-1)+2 \cdot ax^(2-1)+0\\\implies \frac{\text{d}y}{\text{d}x}& = 3x^2+2ax\end{aligned}`

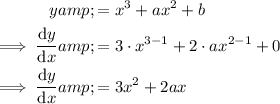
Set the differentiated function to zero:

`\begin{aligned} \frac{\text{d}y}{\text{d}x}& =0\\ \implies 3x^2+2ax & = 0 \\ \implies x(3x+2a)&=0\end{aligned}`

Therefore the stationary points occur when:

`\implies x=0`

`\implies 3x+2a=0 \implies x=-(2)/(3)a`

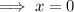
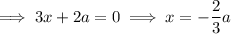
There is a stationary point at (4, -11), therefore substitute x = 4 into the expression for x and solve for a:

`\begin{aligned}x & = 4\\\implies -(2)/(3)a & = 4\\a & = -(3)/(2)(4)\\a & = -6\end{aligned}`

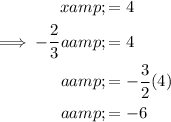
Substitute the found value of a and the point (4, -11) into the function and solve for b:

`\begin{aligned}y & = x^3 + ax^2 + b\\\implies -11 & = (4)^3+(-6)(4)^2+b\\ -11 & = 64-96+b\\b & = -11-64+96\\ b & = 21\end{aligned}`

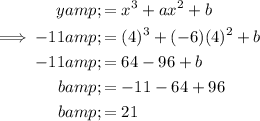
Therefore, the function is:

`\boxed{y=x^3-6x^2+21}`

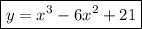
The other stationary point is when x = 0. Therefore, to find the coordinates of this point, substitute x = 0 into the function:

`\begin{aligned}y & =x^3-6x^2+21\\x=0\implies y & =(0)^3-6(0)^2+21\\ y & =21 \end{aligned}`

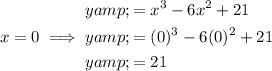
Therefore, the coordinates of the other stationary point are (0, 21).

To determine if a stationary point is minimum or maximum, differentiate the function again:

`\begin{aligned}y & =x^3-6x^2+21\\\implies \frac{\text{d}y}{\text{d}x} & = 3x^2-12x\\\implies \frac{\text{d}^2y}{\text{d}x^2} & = 6x-12\\\end{aligned}`

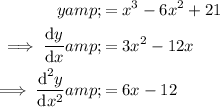
Substitute the x-coordinate of the stationary point into the second derivative:

`\begin{aligned} \frac{\text{d}^2y}{\text{d}x^2} & = 6x-12\\x=0 \implies \frac{\text{d}^2y}{\text{d}x^2} & = 6(0)-12= -12 \\\end{aligned}`

`\textsf{As $\frac{\text{d}^2y}{\text{d}x^2} < 0$, stationary point $(0,21)$ is a maximum.}`

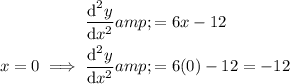
Differentiation Rules

`\boxed{\begin{minipage}{4.8 cm}\underline{Differentiating $ax^n$}\\\\If $y=ax^n$, then $\frac{\text{d}y}{\text{d}x}=nax^(n-1)$\\\end{minipage}}`

`\boxed{\begin{minipage}{4cm}\underline{Differentiating a constant}\\\\If $y=a$, then $\frac{\text{d}y}{\text{d}x}=0$\\\end{minipage}}`