The square root of 1 is 1, but the square root of 18 needs to be explored. 18 is 9*2, and 9 is a perfect square. So let's rewrite our original problem using that fact:
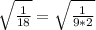
. We can pull out the square root of 1 as 1 and the square root of 9 as 3, leaving the 2 behind:

. And there you go!