Question

Write the expression in interval notation

Answer 1

If we have a less than (or less than or equal to) sign with an absolute value, that indicates a conjunction where values can be expressed as an interval. The absolute value will be less than the number to the right, but it will also at the same time be greater than the negative of the number to the right. Don't forget that absolute value is a distance measurement, and the distance from the origin to -4 is 4 units, just like the distance from the origin to +4 is 4 units. Our particular absolute value will look like this:
`\left[\begin{array}{ccc}x-6\end{array}\right] \leq 4` and
`\left[\begin{array}{ccc}x-6\end{array}\right] \geq -4`. Solving the first absolute value we have that
`x \leq 10` and the second one is
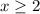
`x \geq 2`. In interval notation we need to put x between the 2 inequality signs, which will look like this:
`2 \leq x \leq 10`. And there you go!