Question

The endpoints of JK are J(–25, 10) and K(5, –20). What is the y-coordinate of point L, which divides JK into a 7:3 ratio

Answer 1

We are looking the coordinate that divides that segment into a 7/3 ratio. If we are dividing that segment and looking for a particular coordinate, we must first divide the segment into 7+3 segmments, or 10 equal length segments. When we plot point L on the segment in a 7/3 ratio, notice that L is 7/10 of the way from J to K. That ratio is k, found by writing the numerator of our ratio over the sum of the numerator and denominator. Our k then is 7 over 7+3, so k = 7/10. Now we will find the rise and the run (aka the slope) of the line.
`m= (-20-10)/(5-(-25)) = (-30)/(30)`. We have a rise value of -30 and a run value of 30. The point L's coordinate are found in this formula:
`(x,y)=( x_(1)+k(run), y_(1) +k(rise))`. x1 and y1 are found in the first coordinate, point J (-25, 10). Filling in accordingly, we have
`(x,y)=(-25+ (7)/(10)(30),10+ (7)/(10)(-30))` which simplifies to
`(x,y)=(-25+21,10-21)`. Those coordinates then of L are (-4, -11)

Answer 2

Answer: The y co-ordinate of the point L is -11.

Step-by-step explanation: Given that the co-ordinates of the endpoints of the line segment JK are J(–25, 10) and K(5, –20).

We are to find the y co-ordinate of he point L that divides the line segment JK in the ratio 7 : 3.

We know that

the co-ordinates of a point that divides the line segment with endpoints (a, b) and (c, d) in the ratio m : n are given by

`\left((mc+na)/(m+n),(md+nb)/(m+n)\right)\\\\\\\Rightarrow \textup{y co-ordinate}=(md+nb)/(m+n).`

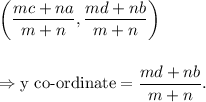
Therefore, the y co-ordinate of the point L will be

`y=(7* (-20)+3*10)/(7+3)=(-140+30)/(10)=(-110)/(10)=-11.`

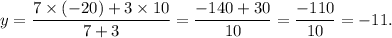
Thus, the y co-ordinate of the point L is -11.