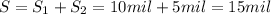Question

Suppose you are driving to school from your home.During the first 15 minutes you drove with an average speed of 40 miles/hour.Then you slow down to 20 miles/hour.If you take 30 minutes to reach school then how far is the school from your home.

Answer 1

We can solve the problem by using the basic relationship between space (S), time (t) and velocity (v) of the uniform motion:

`v= (S)/(t)`

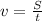
The duration of the first part of the trip is

`t_1 = 15 min= (1)/(4) h`
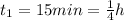
And the velocity is

`v_1=40 mil/h`
So we can find the distance covered during the first part of the trip by rearranging the previous equation:

`S_1 = v_1 t_1 =(40 mil/h)( (1)/(4)h)=10 mil`

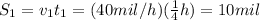
The duration of the second part of the trip is 15 minutes (because the duration of the total trip is 30 minutes, and the duration of the first part is 15 minutes), so

`t_2 = 15 min = (1)/(4)h`
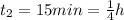
and the velocity is

`v_2 =20 mil/h`
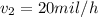
Therefore, the distance covered in this second part of the trip is

`S_2 = v_2 t_2 = (20 mil/h)( (1)/(4)h)=5 mil`

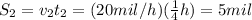
So, the total distance between the home and the school is

`S=S_1 +S_2 = 10 mil+5 mil=15 mil`