Question

How do I figure this out

Answer 1

`27^{(1)/(3)} \quad 125^{(2)/(3)} \quad 9^{(3)/(2)}`

Explanation:

Rewrite 9 as 3²:

`\implies 9^{(3)/(2)}=\left(3^2\right)^{(3)/(2)}`

`\textsf{Apply exponent rule} \quad (a^b)^c=a^(bc):`

`\implies 3^{(2 \cdot (3)/(2))}=3^3`

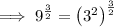
Therefore:

`\implies 3^3= 3 \cdot 3 \cdot 3=27`

---------------------------------------------------------

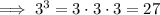
Rewrite 27 as 3³:

`\implies 27^{(1)/(3)}=\left(3^3\right)^{(1)/(3)}`

`\textsf{Apply exponent rule} \quad (a^b)^c=a^(bc):`

`\implies 3^{(3 \cdot (1)/(3))}=3^1`

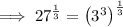
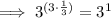
Therefore:

`\implies 3^1=3`

---------------------------------------------------------

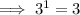
Rewrite 125 as 5³:

`\implies 125^{(2)/(3)}=\left(5^3\right)^{(2)/(3)}`

`\textsf{Apply exponent rule} \quad (a^b)^c=a^(bc):`

`\implies 5^{(3 \cdot (2)/(3))}=5^2`

Therefore:

`\implies 5^2=5 \cdot 5=25`

---------------------------------------------------------

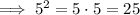
Solution

In order, from smallest to largest:

`27^{(1)/(3)} \quad 125^{(2)/(3)} \quad 9^{(3)/(2)}`