Question

Find the sum of a finite geometric series.

Amanda teaches the art of quilling to 4 students. These students each teach the art of quilling to 4 other students. If this process continues for 5 generations, ____ people will know the art of quilling.

Please fill in the blank.

Answer 1

1364 people

Explanation:

Answer 2

assuming you meant Quilting.

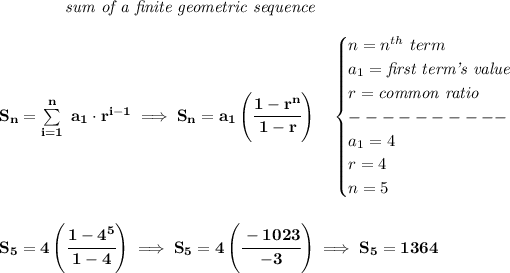
since there are 4 students in her class, that'd be the first term, and then the 4 students each teaches another 4 students, and so on, so the amount of folks who'd know quilting will be

4, 16, 64, ....

where the "common ratio" r = 4, and a₁=4


`\bf \qquad \qquad \textit{sum of a finite geometric sequence} \\\\ S_n=\sum\limits_(i=1)^(n)\ a_1\cdot r^(i-1)\implies S_n=a_1\left( \cfrac{1-r^n}{1-r} \right)\quad \begin{cases} n=n^(th)\ term\\ a_1=\textit{first term's value}\\ r=\textit{common ratio}\\ ----------\\ a_1=4\\ r=4\\ n=5 \end{cases} \\\\\\ S_5=4\left( \cfrac{1-4^5}{1-4} \right)\implies S_5=4\left( \cfrac{-1023}{-3} \right)\implies S_5=1364`