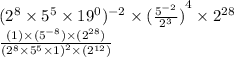
First, we see that everything is multiplied or divided, so we can combine everything into one large fraction.
We can recognize that anything with the exponent 0 equals 1, so 19^0=1.
Since (2^8 × 5^-8 × 19^0) is to the power of -2, we can change the -2 to a 2 and put it in the denominator.
When 5^-2 and 2^3 are to the power of 4, we multiply the exponents. -2 × 4 = -8 and 3 × 4 = 12, so we put 5^-8 in the numerator, and 2^12 In the denominator.
2^28 goes in the numerator.
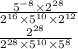
We can get rid of the 1s, since multiplying by 1 does not change any number.
We can factor in the exponent of 2 into (2^8 × 5^5) by multiplying 8 × 2 = 16 and 5 × 2 = 10. The new values are 2^16 and 5^10.
We can move the 5^-8 to the denominator and change it to 5^8.
We van combine 2^16 and 2^12 by adding the exponents.

We can see that there is 2^28 in the numerator, and 2^28 in the denominator, so we can subtract them. Since 28 - 28 = 0, we can eliminate both of them.
We can combine 5^10 and 5^8 to get 5^18.
1 over 5^18 is also equal to 5^-18.
Depending on what your teacher is looking for, the answer is one of those two.