Question

HELP ASAP!

A gardener wants to create a rectangular vegetable garden in a backyard. He wants it to have a total area of 112 square feet, and it should be 14 feet longer than it is wide. What dimensions should he use for the vegetable garden? Round to the nearest hundredth of a foot.

12.69 feet by 26.69 feet

10.58 feet by 24.58 feet

5.69 feet by 19.69 feet

3.58 feet by 17.58 feet

Thank you!

Answer 1

It is C because  5.69 multiplied by 19.69 equals 112.0361 Square Foot

Answer 2

5.69 feet by 19.69 feet

Explanation:

Given : A gardener wants to create a rectangular vegetable garden in a backyard. He wants it to have a total area of 112 square feet, and it should be 14 feet longer than it is wide.

Let x be the breadth of the rectangular vegetable garden

So, length of the rectangular vegetable garden = ( x + 14 ) feet

To find: dimensions that a gardener should use for the vegetable garden

Solution :

We know that area of the rectangle is equal to length ( l ) × breadth ( b )

Area of the rectangular garden = 112 square feet

According to question,

`x(x+14)=112\Rightarrow x^2+14x-112=0`

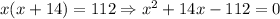
In order to find roots, use quadratic formula which states that for equation
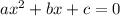`ax^2+bx+c=0`, roots are
`(-b\pm √(b^2-4ac))/(2a)`

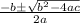
Therefore, for equation
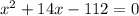`x^2+14x-112=0`, roots are
`(-14\pm √(196+448))/(2)=(-14\pm √(644))/(2)`

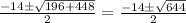
Being a dimension, breadth can not be negative, so x =
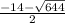`(-14- √(644))/(2)` is rejected

Therefore,

`x=(-14+ √(644))/(2)=5.69\Rightarrow l = x+14=5.69+14=19.69`

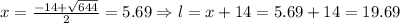
So, length = 19.69 feet and breadth = 5.69 feet