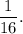Question

Enter the correct value so that each expression is a perfect-square trinomial.

x2 + 1/2x + ___

Answer 1

Answer: The required correct value is
`(1)/(16).`

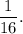
Step-by-step explanation: We are given to find the correct value so that the following expression is a perfect square trinomial :

`E:x^2+(1)/(2)x+?`

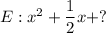
Let the required number be represented by p.

Then, we have

`x^2+(1)/(2)x+p\\\\\\=x^2+2* x* (1)/(4)+\left((1)/(4)\right)^2+p-\left((1)/(4)\right)^2\\\\\\=\left(x+(1)/(4)\right)^2+p-(1)/(16).`

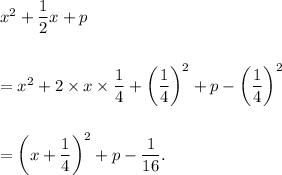
So, to make the given expression a perfect square trinomial, we must have

`p-(1)/(16)=0\\\\\\\Rightarrow p=(1)/(16).`

Thus, the required correct value is
`(1)/(16).`

Answer 2

To solve this problem you must apply the proccedure shown below.
1. By definition, a perfect square trinomial has the following form:

`a^(2)+ 2ab+b^(2)`
2. Therefore, you must divide the middle term by 2 and square the result, as following:

`(1/2)/2=(1/4)^(2)=1/16`
3. As you can see,
`b^(2)=1/16`, so, you can rewrite it as below:

`(x+1/16)^(2)`
The answer is:
`1/16`