Answer:

Explanation:
We are given the following information in the question:
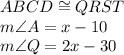
Since,quadrilateral ABCD is congruent to the quadrilateral QRST, then by the property of congruency, we can write,
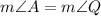
Equating the value of the two angles, we get,
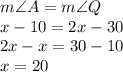
Putting the value of x to obtain measure of angle A.
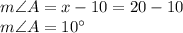
Thus, the measure of angle A is 10 degrees.