Answer:
the volume of the rubber ball when the radius equals
 feet
feet
Explanation:
see the attached figure to better understand the problem
we know that
The volume of a sphere (rubber ball) is equal to
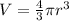
where
r is the radius of the sphere (rubber ball)
The volume in function notation is equal to
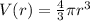
in this problem we have
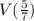
that means
The radius of the rubber ball is
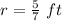
and
The volume of the rubber ball is
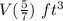
therefore
the answer is the option B
the volume of the rubber ball when the radius equals
 feet
feet