Question

Can someone please help me with this? i still have 2 more pages to do and I'm stressed out of my mind I honestly just wanna pass Math so I can move on?? So can someone help me please ?

Answer 1

1. First we are going to find the vertex of the quadratic function
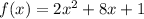
`f(x)=2x^2+8x+1`. To do it, we are going to use the vertex formula. For a quadratic function of the form
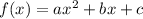
`f(x)=ax^2+bx +c`, its vertex
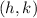
`(h,k)` is given by the formula
`h= (-b)/(2a)`;
`k=f(h)`.

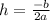
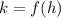
We can infer from our problem that
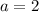
`a=2` and
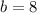
`b=8`, sol lets replace the values in our formula:

`h= (-8)/(2(2))`

`h= (-8)/(4)`

`h=-2`

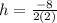
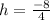
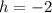
Now, to find
`k`, we are going to evaluate the function at
`h`. In other words, we are going to replace
`x` with -2 in the function:

`k=f(-2)=2(-2)^2+8(-2)+1`

`k=f(-2)=2(4)-16+1`

`k=f(-2)=8-16+1`

`k=f(-2)=-7`

`k=-7`
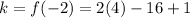
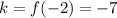
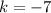
So, our first point, the vertex
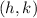
`(h,k)` of the parabola, is the point
`(-2,-7)`.

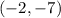
To find our second point, we are going to find the y-intercept of the parabola. To do it we are going to evaluate the function at zero; in other words, we are going to replace
`x` with 0:

`f(x)=2x^2+8x+1`

`f(0)=2(0)^2+(0)x+1`

`f(0)=1`
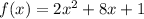
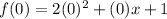
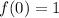
So, our second point, the y-intercept of the parabola, is the point (0,1)

We can conclude that using the vertex (-2,-7) and a second point we can graph
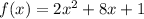
`f(x)=2x^2+8x+1` as shown in picture 1.

2. The vertex form of a quadratic function is given by the formula:
`f(x)=a(x-h)^2+k`
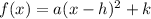
where

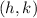
`(h,k)` is the vertex of the parabola.

We know from our previous point how to find the vertex of a parabola.
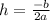
`h= (-b)/(2a)` and
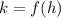
`k=f(h)`, so lets find the vertex of the parabola
`f(x)=x^2+6x+13`.

`a=1`

`b=6`

`h= (-6)/(2(1))`

`h=-3`

`k=f(-3)=(-3)^2+6(-3)+13`

`k=4`

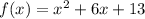
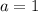
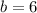
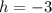
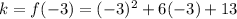
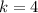
Now we can use our formula to convert the quadratic function to vertex form:

`f(x)=a(x-h)^2+k`

`f(x)=1(x-(-3))^2+4`

`f(x)=(x+3)^2+4`

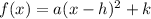
We can conclude that the vertex form of the quadratic function is
`f(x)=(x+3)^2+4`.

3. Remember that the x-intercepts of a quadratic function are the zeros of the function. To find the zeros of a quadratic function, we just need to set the function equal to zero (replace
`f(x)` with zero) and solve for
`x`.

`f(x)=x^2+4x-60`

`0=x^2+4x-60`

`x^2+4x-60=0`
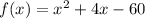
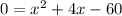
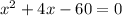
To solve for
`x`, we need to factor our quadratic first. To do it, we are going to find two numbers that not only add up to be equal 4 but also multiply to be equal -60; those numbers are -6 and 10.

`(x-6)(x+10)=0`
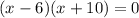
Now, to find the zeros, we just need to set each factor equal to zero and solve for
`x`.

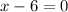
`x-6=0` and
`x+10=0`

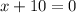
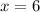
`x=6` and
`x=-10`

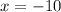We can conclude that the x-intercepts of the quadratic function
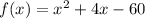`f(x)=x^2+4x-60` are the points (0,6) and (0,-10).

4. To solve this, we are going to use function transformations and/or a graphic utility.
Function transformations.
- Translations:
We can move the graph of the function up or down by adding a constant
`c` to the y-value. If
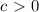
`c\ \textgreater \ 0`, the graph moves up; if
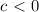
`c\ \textless \ 0`, the graph moves down.

- We can move the graph of the function left or right by adding a constant
`c` to the x-value. If
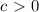
`c\ \textgreater \ 0`, the graph moves left; if
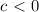
`c\ \textless \ 0`, the graph moves right.

- Stretch and compression:
We can stretch or compress in the y-direction by multiplying the function by a constant
`c`. If
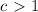
`c\ \textgreater \ 1`, we compress the graph of the function in the y-direction; if
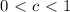
`0\ \textless \ c\ \textless \ 1`, we stretch the graph of the function in the y-direction.

We can stretch or compress in the x-direction by multiplying
`x` by a constant
`c`. If
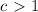
`c\ \textgreater \ 1`, we compress the graph of the function in the x-direction; if
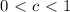
`0\ \textless \ c\ \textless \ 1`, we stretch the graph of the function in the x-direction.

a. The
`c` value of
`f(x)` is 2; the
`c` value of
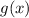
`g(x)` is -3. Since
`c` is added to the whole function (y-value), we have an up/down translation. To find the translation we are going to ask ourselves how much should we subtract to 2 to get -3?

`c+2=-3`

`c=-5`

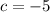
Since
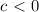
`c\ \textless \ 0`, we can conclude that the correct answer is: It is translated down 5 units.

b. Using a graphing utility to plot both functions (picture 2), we realize that
`g(x)` is 1 unit to the left of
`f(x)`

We can conclude that the correct answer is: It is translated left 1 unit.

c. Here we have that
`g(x)` is
`f(x)` multiplied by the constant term 2. Remember that We can stretch or compress in the y-direction (vertically) by multiplying the function by a constant
`c`.

Since
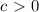
`c\ \textgreater \ 0`, we can conclude that the correct answer is: It is stretched vertically by a factor of 2.