Answer:
(-4,-8)
Explanation:
Given is a picture with a point R and a line PQ
We have to find the point out of the 4 options lying on the perpendicular line to PQ through R
R is the point (4,2)
P is (-6,4) and Q is (4,-4)
Slope of PQ =
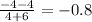
Slope of perpendicular line would be =
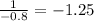
Equation of the line through R with slope -1.25 is
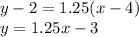
Out of the four options,
only (-4,-8) satisfies
because
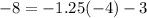
so answer is (-4,-8)