Question

Peter writes a blog covering news from his local area. He placed a survey on his blog asking whether users desired a change to his blog’s design. A total of 240 users took the survey on a day when the website had 2,975 visitors. Peter found that 55% of those surveyed were in favor of changing the design. Assuming a 90% confidence level, which of the following statements holds true?

A: As the sample size is appropriately large, the margin of error is ±0.053.
B:As the sample size is appropriately large, the margin of error is ±0.063.
C:As the sample size is too small, the margin of error cannot be trusted.
D:As the sample size is too small, the margin of error is ±0.053.

Answer 1

A: As the sample size is appropriately large, the margin of error is ±0.053.

correct

Answer 2

Option A is correct

Explanation:

Given that Peter placed a survey on his blog asking whether users desired a changed to his blog's design.

A total of 240 users took the survey on a day when there were 2975 visitors

Sample proportion p= 55%=0.55

q=1-0.55 =0.45

n =240

std error =
`\sqrt{(pq)/(n) } =0.0321`

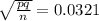
For 90% z value = 1.645

Margin of error =±1.645(0.0032)

=0.0528

=±0.053

A: As the sample size is appropriately large, the margin of error is ±0.053.

D is not right because sample size is not too small, a sample size more than 30 cannot be considered as too small. Also out of 2975 visitors, 240 took survey which seems reasonable size.