Answer with explanation:
For, two Events, A and B
P(A)=0.3 , P(B)=0.8 , and P(A ∩ B)=0.24
⇒P(A)×P(B)= 0.8 × 0.3= 0.24
⇒P(A ∩ B)=P(A)×P(B)
Hence the Events are Independent.
Now, by looking at the options
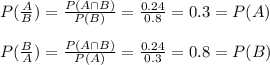
The events are Independent.
None, of the option are true if the two events are independent.