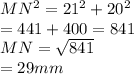Question

In the pulley system shown in this figure, MQ = 30 mm, NP = 10 mm, and QP = 21 mm. Find MN.

Answer 1

MN =29 mm

Explanation:

Two circles are there with radius 30 and 10 mm.

Common tangent has length as 21.

We have to find the distance between the centres.

If we draw a line NT parallel to PQ meeting QM at T

then we find that QTNP is a rectangle.

Hence NT=21 mm

NTM would be right triangle right angled at T, having hypotenuse as MN

one side =21 and other side = 30-10 =20

Using Pythagorean theorem,

we get

`MN^(2) =21^(2) +20^(2) \\=441+400 =841\\MN =√(841) \\=29 mm`

Answer 2

the picture in the attached figure

step 1
Draw a line PT parallel to MN such that T lies on MQ
see the attached figure N 2
Then
TQ = MQ - NP = 30-10 = 20 mm
TP=MN

step 2
in the right triangle PTQ
TQ=20 mm
QP=21 mm
TP=?

applying the Pythagoras Theorem
TP²=TQ²+QP²-----> 20²+21²-----> 841
TP=√841------> TP=29 mm
MN=TP----------> MN=29 mm

the answer is
MN=29 mm