You need to use the law of sines.
First, we find the measures of the angles x.
x + x + 100 = 180
2x + 100 = 180
2x = 80
x = 40
Now we set up an equation based on the law of sines.
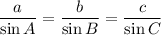
We know one angle (100 deg) and its opposite side (750,000 miles).
Then we know another angle (40 deg) and need to find the opposite side (d).
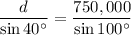
Now we solve for d. Multiply both sides by sin 40.
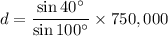
Answer: The first choice.