Question

Which line is perpendicular to a line that has a slope of -5/6?

Answer 1

The answer is B.) Line LM

Hope this helps!!

Answer 2

Line LM is perpendicular to line AB. It's equation is 5y - 6x = 15

Explanation:

Given: Slope of line, AB
`(-5)/(6)`

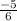
To find: A line which is perpendicular to given line AB

We know that if two lines are perpendicular than product of their slope is -1.

Let slope of required line is m then by using given condition we get,

`m*(-5)/(6)\,=\,-1`

`m\,=\,(6)/(5)`

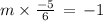
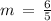
Now we check slope of each and every line and matches with value of m.

using two point we find slope.

formula for slope,

`Slope\,=\,(y_2-y_1)/(x_2-x_1)`

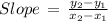
Coordinates of Given points are P( -5 , 4 ) , Q( 0 , -2 ) , J( -6 , 1 ) , K( 0 , -4 ) ,

L( -5 , -3 ) , M( 0 , 3 ) , N( -6 , -5 ) and O( 0 , 0 )

Slope of line PQ =
`(-2-4)/(0-(-5))\:=\:(-6)/(5))`

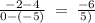
Slope line JL =
`(-4-1)/(0-(-6))\:=\:(-5)/(6))`

Slope line LM =
`(3-(-3))/(0-(-5))\:=\:(6)/(5))`

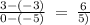
Slope line NO =
`(0-(-5))/(0-(-6))\:=\:(5)/(6))`

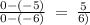
Thus, By comparing with above slope.

LM is our required line which is perpendicular to given Line AB.

For equation we use point-slope form,

Equation line LM

`(y-y_1)=m*(x-x_1)`

`(y-3)=(6)/(5)*(x-0)`

`5*(y-3)=6*(x-0)`

`5y-15=6x`

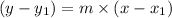
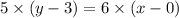
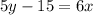
5y - 6x = 15

Therefore, Line LM is perpendicular to line AB. It's equation is 5y - 6x = 15