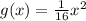Question

If you horizontally stretch the quadratic parent function f(x) = x^2 by a factor of 4 what’s the equation of the new function

Answer 1

f'(x)=(x/4)^2 or (x^2)/16

Answer 2

Horizontal stretch:

If a parent function y = f(x) ,

Replace x with
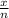`(x)/(n)` result in horizontal stretch by a factor of n.

then a new function become
`g(x) = f((x)/(n))`

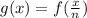
As per the statement:

Horizontally stretch the quadratic parent function
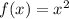`f(x) = x^2` by a factor of 4 .

By definition of Horizontal stretch:

A equation of new function become:

`g(x) = f((x)/(4))=((x)/(4))^2=(1)/(16)x^2`

therefore, a equation of the new function is,
`g(x) = (1)/(16)x^2`