The one which lies in Quadrant II is
D(-n,n). Quadrant II has positive y coordinate y and negative x coordinate.
Here's a way to find the midpoint
x midpoint =
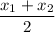
y midpoint =
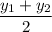
Find x midpoint, plug in the numbers
x midpoint =
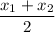
x midpoint =
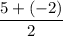
x midpoint =

x midpoint = 1.5
Find y midpoint, plug in the numbers
y midpoint =
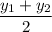
y midpoint =
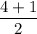
y midpoint =

y midpoint = 2.5
The midpoint of the line segment is (1.5, 2.5)