Question

For the function f(x)= 1/x-2 , whose graph is shown below. what is the relative value of F(x) when the value of x is close to 2?

A.either a very large positive or very large negative number
B.either a very small positive or very small negative number
C.only a very small positive number
D.a very large positive number
E.only a very large negative number

Answer 1

A

Explanation:

This is a reciprocal function graph with an asymptote at x = 2.

An asymptote is a line that the graph comes close and close to, but never touches. This is the "line" where the function is not defined.

The equation is
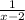`(1)/(x-2)`, so u can see that we can't use the value x = 2 because it will make the denominator 0, hence x = 2 is the asymptote.

Now, if you look at the graph, when you approach x = 2 FROM THE RIGHT SIDE, the value of f(x) gets very large, going towards positive infinity.

When you approach x = 2 FROM THE LEFT SIDE, the value of f(x) gets very large, going towards negative infinity.

Answer choice A is right.

Answer 2

The graph shows the correct choice to be
A. either a very large positive or very large negative number

_____
Which of those it is depends on whether x is slightly more or slightly less than 2.