Question

9/y+1=18/54
cross multiply

Answer 1

Cross multiplying can be known shown like this. a/b=c/d ad=bc

We have a similar situation here. In this case, 9 is a, y+1 is b, 18 is c and 54 is d. We can, first of all, simplify 18/54 to 1/3, so now we have 9/y+1=1/3.
Now we can use the formula above to solve for y.
So,
9*3=(y+1)*1
27=y+1
Subtract 1 from both sides
y=26

Answer 2

If all you want to do is "cross multiply," you multiply the equation by the product of the denominators.


`(9\cdot 54\cdot (y+1))/(y+1)=(18\cdot 54\cdot (y+1))/(54)`


`9\cdot 54=18\cdot (y+1)`
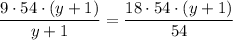
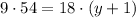
The denominator on each side cancels the corresponding factor in the numerator so the appearance is that you've multiplied the numerator on one side by the denominator on the other side. That is why this is called "cross multiplying." (The rules of equality require you do the same thing to both sides of the equation. What you are actually doing is multiplying both sides by the same product of denominators and taking advantage of the cancellations that occur.)

In this case, you can work with smaller numbers if you reduce the fraction 18/54 to 1/3 first. Then the result of "cross multiplying" is
9·3 = y+1

_____
You can finish the solution by subtracting 1.
26 = y