Question

NEED ANSWERS ASAP!! Help Pls I’d really appreciate it :)

Answer 1

Hello,
Please, see the detailed solution in the attached files.
Thanks.

Answer 2

Question 23:

The area of the shaded portion of the circle = area of the arc - area of the triangle
Area of arc =
`(72)/(360) * \pi r^2 = (72)/(360)* \pi * 5^2 = (72*25)/(360) \pi = 5 \pi`
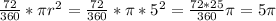
Area of triangle = 0.5 * base * height = 0.5 (2.9 + 2.9) * 4 = 11.6
Area of the shaded portion = (5π - 11.6) ft²
So, the correct answer is option A
=================================================
Question 24:
As shown in the problem the two congruent shaded figures have the following properties:
1. Each figure has four sides.
2. For each figure, each two opposite sides are parallel.

So, The correct statements are D and E
D. The figures are parallelograms because their opposite sides are parallel.
E. The figures are quadrilaterals because each has four sides.
=================================================
Question 25:
The length of the line segment GH will be calculated as following:
Δ CEG is aright triangle at E
So the hypotenuse is CG = √(CE² + EG²)
∴ CG = √(9² + 12²) = √225 = 15 →→ (1)
For the circle C
CH = CE = radius of the circle = 9 →→(2)
From (1) and (2)
∴ GH = CG - CH = 15 - 9 = 6

So, the correct answer is option D. 6 units
=================================================
Question 26:
For the right cylinder
let the height of the cylinder = h
and let the radius of the base = r
so, the volume = π r² h

Given that: the height of the cylinder is 3 times the radius of the base∴ h = 3 r
Given the volume = 24π
∴ volume = π r² h = π r² * ( 3r ) = 3π r³
3π r³ = 24 π ⇒⇒⇒ divide both sides over 3π
∴ r³ = (24π)/(3π) = 8
∴ r = ∛8 = 2
∴ h = 3r = 3 * 2 = 6

So, the correct answer is option C. 6 units