Please HELP ME!!!!!! No one is helping me :(

Question

asked May 14, 2019 199k views

1 Answer

Greg Ostry · Answer 1 · 2019-05-19T12:30:57+0000

Obviously, you're solving for e. Divide both sides by 9f to get
e^(2)= (49)/(9f)

. Undo the square on the e by taking the square root of both sides, leaving you with
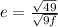
e= ( √(49) )/( √(9f) )

. Both 49 and 9 are perfect squares, so simplify accordingly to get that
e=+/- (7)/(3 √(f) )

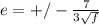
. If you have to rationalize the denomiator, then your final answer would be
e=+/- (7 √(f) )/(3f)