Answer:
Option B and D are correct
 or
or

Explanation:
Using exponent rule:
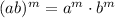
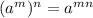
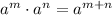
Given the expression:
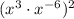 ....[1]
....[1]
Apply the exponent rule:
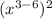

We can write the given expression as:
Apply the exponent rule in [1]:

⇒

Therefore, the first step in simplifying this expression are:
 or
or
