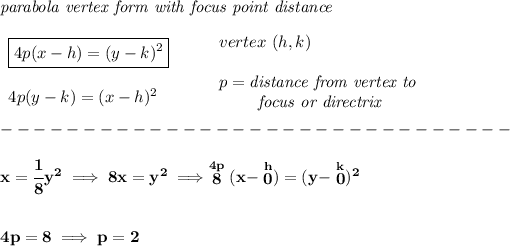
first off, notice that the equation has a squared variable of "y", meaning the parabola is a horizontal one, the "p" distance is 2, so "p" is positive, and the vertex is at 0,0, namely the origin.
if we move from the origin 2 units to the right, we'll land on the focus point at (2,0), and if we move 2 units in the opposite direction, we'll land on the directrix, check the picture below.