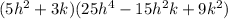Question

A cube with side length 5h^2is stacked on another cube with side length 3k. What is the total volume of the cubes in factored form?

Answer 1

( 5h2 + 3k ) ( 25h4 - 15h2k + 9k2 )

Explanation:

Answer 2

we know that

The volume of the first cube is
(5h^2)^3
while
the volume of the second cube is
(3k)^3
so
their total volume is
(5h^2)^3 + (3k)^3

We can use the special formula for factoring a sum of two cubes:
x^3 + y^3 = (x + y)(x^2 - xy + y^2)
(5h^2)^3 + (3k)^3 = (5h^2 + 3k)((5h^2)^2 - (5h^2)(3k) + (3k)^2)
= (5h^2 + 3k)(25h^4 - 15(h^2)(k) + 9k^2)

the answer is

`(5 h^(2) +3k)(25 h^(4)-15 h^(2)k+9k^(2) )`