Answer:
β≈13.83°
Explanation:
Hi there!
We have three sides and no angle measure displayed.
And when we have a not rectangular triangle and more than one angle information, then certainly we can apply the Law of Sines.
But all we want to find it here is one information, one angle. The least measure angle. So we need then the Law of Cosines.
And the question wants us to find just this angle.
Therefore, the choice is the Law of Cosines.
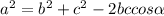
Well, the question wants the angle with the least measure, hence the angle opposed to the least side, as the least side is the side opposed to B, a little adjustment has to be made turning the Law of Cosines to this version:
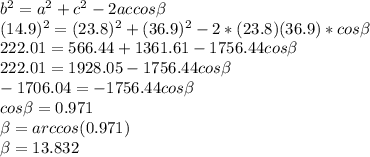
β≈13.83°