This is a problem of
system of linear equations in two variables. This concept of mathematics is very useful in real-life problems. You can solve a system by finding the solution. A
solution of a system are the values of the variables that satisfies each equation in the system. So by knowing these concepts we can get a system of equation by analyzing each statement step by step, that is:
1. Alice has a total of 12 dimes and nickels.
Let the variables:
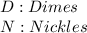
1st. Equation:
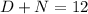 2. She has 2 more nickels than dimes
2. She has 2 more nickels than dimes
2nd Equation:
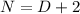
Therefore our system of linear equations is:
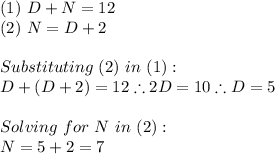
Conclusion:
Alice has 5 dimes and 7 nickels.