point slope form is

where
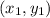
are a point that the line passes through and

is the slope
I guess solve for 1 y and get the constants to the left side3y=x+11divide both sides by 3
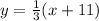
there you have it:
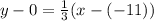
it passes through the point (-11,0) and has a slope of 1/3