Answer:
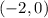 and
and
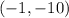 .
.
Explanation:
We have been given a system of equations. We are asked to solve our given system of equations.
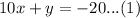

We will use substitution method to solve our given system. From equation (1) we will get,
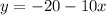
Substituting this value in equation (1) we will get,
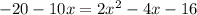
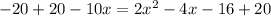
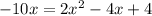
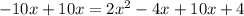
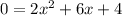
Now we will use quadratic formula to solve for x.
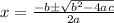
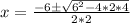
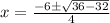
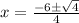
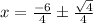
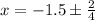

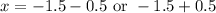
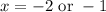
Now to find y values we will substitute
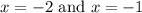 in equation (1) as.
in equation (1) as.
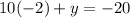
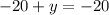
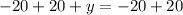

Now, we will substitute
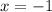 in equation (1) as.
in equation (1) as.
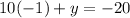
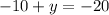
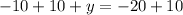
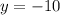
Therefore, there are two solutions for our given system that are
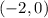 and
and
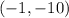 .
.