Question

Write an equation in slope-intercept form of the line perpendicular to the line x + 2y= -2 containing the point (-3, -4)

Answer 1

first off, let's put x + 2y = -2 in slope-intercept form, so can see what its slope is,


`\bf x+2y=-2\implies 2y=-2-x\implies y=\cfrac{-x-2}{2}\implies y=-\cfrac{1}{2}x-1`

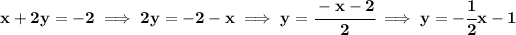
so, as we can see, it has a slope of -1/2.

now, a perpendicular line to that one, will have a negative reciprocal slope,


`\bf \textit{perpendicular, negative-reciprocal slope for}\quad -\cfrac{1}{2}\\\\ negative\implies +\cfrac{1}{ 2}\qquad reciprocal\implies + \cfrac{ 2}{1}\implies 2`

so, we're really looking for a line whose slope is 2 and runs through -3,-4.


`\bf \begin{array}{ccccccccc} &&x_1&&y_1\\ &&(~ -3 &,& -4~) \end{array} \\\\\\ % slope = m slope = m\implies 2 \\\\\\ % point-slope intercept \stackrel{\textit{point-slope form}}{y- y_1= m(x- x_1)}\implies y-(-4)=2[x-(-3)] \\\\\\ y+4=2(x+3)\implies y+4=2x+6\implies y=2x+2`