Question

The step function f(x) is graphed.



What is the value of f(−1)?

Answer 1

Answer: -1

Explanation:

Answer 2

Notice that the first interval [-3,-1) is closed at -3 but open at -1, which means that when the value of
`x` is between -3 and -0.999... the value of
`f(x)` is -3; in other words the function for the first piece is:

`f(x)=-3` ,
`3\leq x\ \textless \ -1`

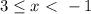
The second interval [-1,1) is closed at -1 but open at 1, which means that when the value of
`x` is between -1 and 0.999.. the value of
`f(x)` is -1; in other words, the function of the second piece is:

`f(x)=-1` ,
`-1 \leq x\ \textless \ 1`

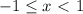We can conclude that the value of
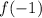`f(-1)` is -1.