Question

Which classification best represents a triangle with side lengths 6cm, 10cm, and 12 cm?

Answer 1

We can sort out this triangle as a scalene triangle, that is, a triangle that has three unequal sides. It is also true that there are no equal angles for this type of triangles. If a scalene triangle has an angle of 90°, then this is called a right triangle. But this is not right triangle. Let's prove it:

We know that the Pythagorean Theorem establishes that:


`c^2=a^2+b^2 \\ where \ a \ and \ b \ are \ the \ sides \ and \\ c \ is \ the \ Hypotenuse \ that \ is \ always \ the \ greatest \ side`

Therefore, we can say that:


`a=10cm \\ b=6cm \\ c=12cm`

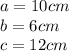
Accordingly:


`12^2 \\eq 10^2+6^2 \therefore \boxed{144 \\eq 136}`

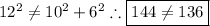In fact, this is not a right triangle.