Question

What is the area of a regular polygon with 16 sides and side length 4 inches

Answer 1

you take 16 and multiply it by 4, giving you the answer that the area is 64

Answer 2

For a better understanding of the solution please find the diagram in the attached file.

The diagram is that of a triangular part of the regular polygon. The triangle
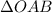`\Delta OAB` is an isosceles triangle because the sides OA and OB are equal. Now, The angle
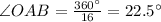`\angle OAB=(360^(\circ))/(16)=22.5^(\circ)`. This is because the angle around the central angle of a polygon is 360 degrees in total.

Now, because
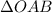`\Delta OAB` is an isosceles triangle, angle OAP will be half of angle OAB. Thus,
`\angle OAP=11.25^(\circ)`.

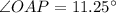AP will be half of AB. Therefore, AP=2.

Now, the apothem, OP can thus be found as:

`(AP)/(OP)=tan(11.25^(\circ))`

`\therefore OP=(AP)/(tan(11.25^(\circ)))=(2)/(tan(11.25^(\circ)))\approx10.1`

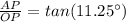
Thus the area of one triangle is
`(1)/(2)* 4* 10.1=20.2`

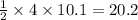
Therefore, the area of all the 16 triangles that make up the regular polygon is:
`16* 20.2=323.2`

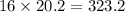
Thus the area of the polygon is 323.2 squared inches.