Use one of your formulas for circles here to find x. Our particular one based on the values given would be x(x+21) = x+1(x+1+14) which simplifies down to x(x+1) = x+1(x+15). We need to distribute now:
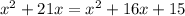
. The nice thing here is that the x-squared terms cancel out when you move one over to combine like terms, leaving us with 5x = 15. Therefore, we find that x = 3.