Suppose
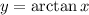
, where
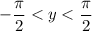
. The restriction on the domain of

is needed for the following step; over this interval, we can properly take the inverse of
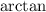
, i.e. take the tangent of both sides:
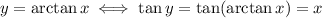
Just to emphasize the point, we can only simplify
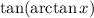
to

as long as
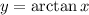
is smaller than
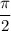
in absolute value.
Now imagine you have a right triangle with a reference angle of

. We're told that
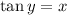
. In this triangle, then, the lengths of its legs occur in a ratio of

. In other words, if one of the legs had length

(the leg adjacent to the angle), then the other must have length

(the leg opposite the angle).
By the Pythagorean theorem, it would follow that the length of the hypotenuse is
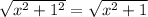
. Then the sine of this angle is
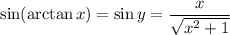
Personally, I wouldn't deem it necessary to rationalize the denominator, but we could go ahead and do it for giggles.
