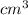Question

A student increases the temperature of a 200 cm3 balloon from 33degrees C to 47degrease C. What will the new volume of the ballon be?

Answer 1

The new volume of balloon when the temperature increases to 47 degree C is 209
`cm^3`

Step-by-step explanation:

According to temperature-volume law, It states that the volume of a gas is directly proportional to the Kelvin temperature, while pressure and amount are held constant.

The equation is
`(V_(1))/(T_(1))=(V_(2))/(T_(2))`

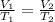
Where V is volume and T is temperature in Kelvins. We know that

V1=
`200 \mathrm{cm}^(3)`

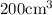
T1=
`33^(\circ) \mathrm{C}` +273=306 K

T2=
`47^(\circ) \mathrm{C}`+273=320 K

To find V2, after Rearranging.

V2=
`(V_(1) T_(2))/(T_(1))`

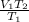
V2 =
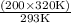`\frac{(200 * 320 \mathrm{K})}{293 \mathrm{K}}`= 209
`cm^3`

Answer 2

The volume is directly related to the temperature of a gas. You can solve this question using the ideal gas equation(specifically Charles' law), but remember to convert the temperature from Celcius to Kelvin.

V1/T1= V2/T2
200 cm3/ (33+273K)= V2/(47+273K)
V2= 200cm3 * 320/306= 209.15cm3